Degrees to Radians

Theory

Radian, a circle measure

One radian is the angle that an arc of 1 unit subtends at the centre of a circle of radius 1 unit.

 

Degrees to radians conversion:  1 degrees = π/180 radians

 

Radians and Degrees

π^c = 180° 

where π^c  = π  radians

Relationship between radian measure and degrees

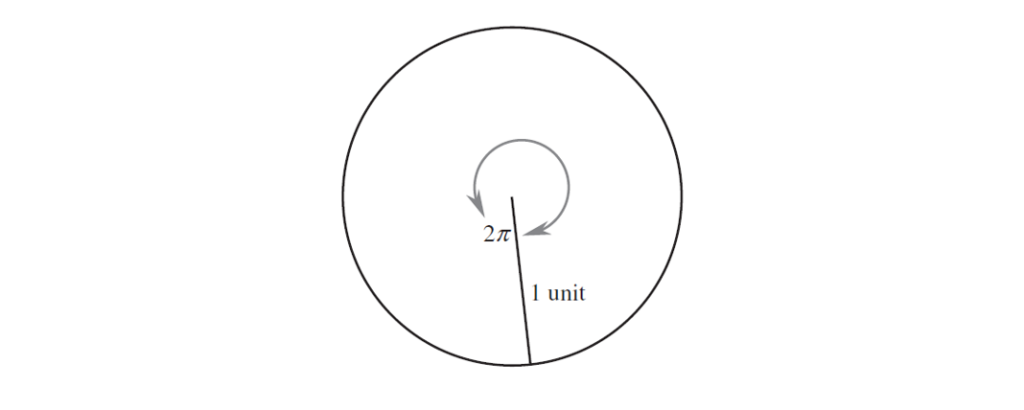
Circumference of the circle with radius 1 unit is given by:

C = 2πr

= 2π(1)

= 2π

T

The arc length of the whole circle is 2π.

 

∴ There are 2π radians in a whole circle.

 

But there are 360° in a whole circle (angle of revolution).

So 2π^c = 360°

π^c = 180°

 

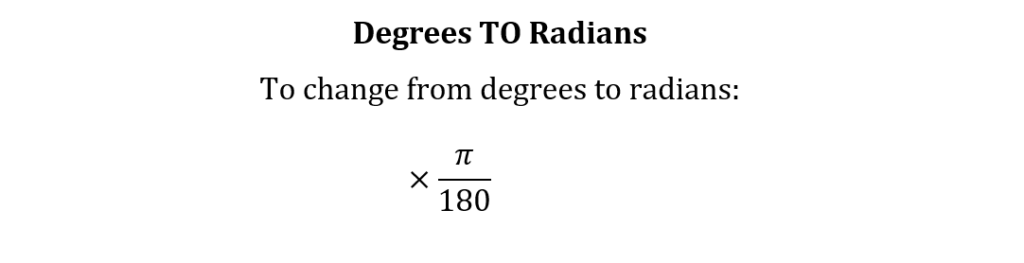
Degrees To Radians

To change from degrees to radians: multiply by π/180

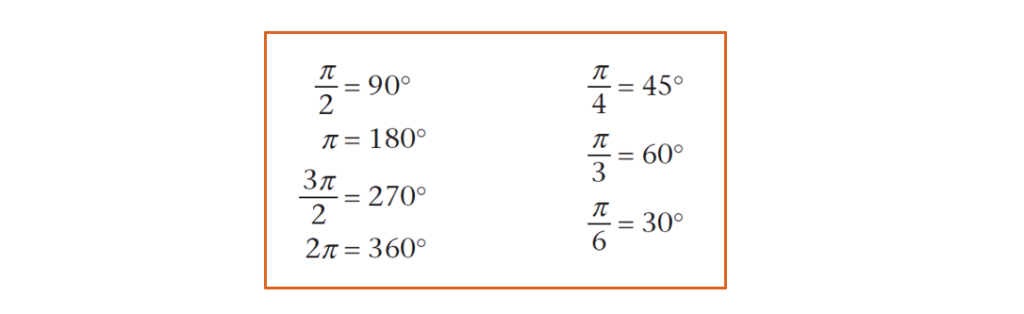
Note: Special measure you will use regularly include -

 

Practice Question

 

1. Convert 150°  into radians.

Solution

1. To convert degrees into radians:

Therefore:

150°=150 x π/180 radians

 

=150π/180 radians

 

 =5π/6 radians